
ZhETF, Vol. 122,
No. 3,
p. 647 (September 2002)
(English translation - JETP,
Vol. 95, No. 3,
p. 560,
September 2002
available online at www.springer.com
)
FRACTAL DIFFUSION IN SMOOTH DYNAMICAL SYSTEMS WITH VIRTUAL INVARIANT CURVES
Chirikov B.V., Vecheslavov V.V.
Received: April 5, 2002
PACS: 05.45.Ac
Preliminary results of extensive numerical experiments with a family of simple models specified by the smooth canonical strongly chaotic 2D map with global virtual invariant curves are presented. We focus on the statistics of the diffusion rate D of individual trajectories for various fixed values of the model perturbation parameters K and d. Our previous conjecture on the fractal statistics determined by the critical structure of both the phase space and the motion is confirmed and studied in some detail. In particular, we find additional characteristics of what we earlier termed the virtual invariant curve diffusion suppression, which is related to a new very specific type of the critical structure. A surprising example of ergodic motion with a «hidden» critical structure strongly affecting the diffusion rate was also encountered. At a weak perturbation (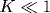 ), we discovered a very peculiar diffusion regime with the diffusion rate D=K2/3 as in the opposite limit of a strong ( ), we discovered a very peculiar diffusion regime with the diffusion rate D=K2/3 as in the opposite limit of a strong (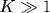 ) uncorrelated perturbation, but in contrast to the latter, the new regime involves strong correlations and exists for a very short time only. We have no definite explanation of such a controversial behavior. ) uncorrelated perturbation, but in contrast to the latter, the new regime involves strong correlations and exists for a very short time only. We have no definite explanation of such a controversial behavior.
|
|